Description
ACCUPLACER Next-Generation Quantitative Reasoning, Algebra, and Statistics
Next-Generation Quantitative Reasoning, Algebra, and Statistics – Question 11 of 20
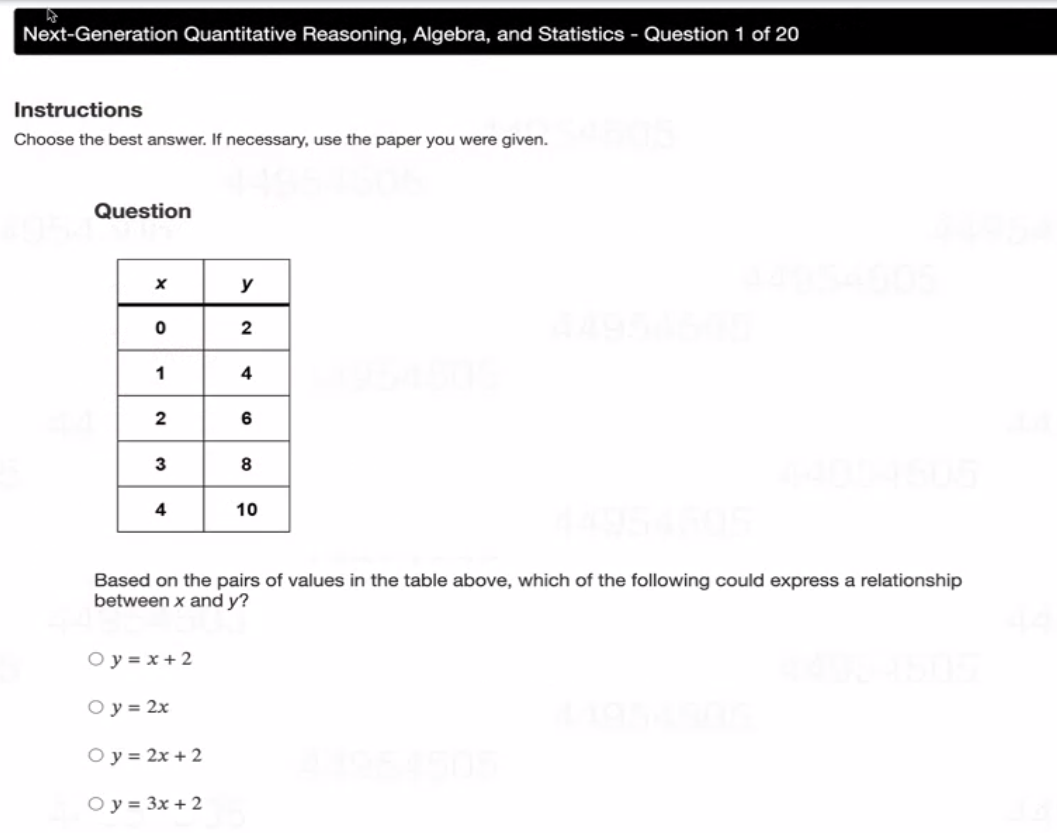
Question: Every month Tanesha pays a fixed fee of $10 to use the parking lot at her workplace. She must also pay $2 each day that she parks her car in the lot. If she parks there xx days in one month, which of the following represents the amount, in dollars, that she must pay for using the parking lot that month?
Options:
- 2x2x
- 12x12x
- 12x\frac{12}{x}
- 10+2×10 + 2x
Answer: The correct answer is 10+2×10 + 2x.
This represents the fixed monthly fee of $10 plus $2 for each day xx that she parks.
Next-Generation Quantitative Reasoning, Algebra, and Statistics – Question 12 of 20
Question: The figure above is a semicircle of radius xx. What is the perimeter of the figure?
Options:
- (π+1)x(\pi + 1)x
- (π+2)x(\pi + 2)x
- (2π+1)x(2\pi + 1)x
- (2π+2)x(2\pi + 2)x
Answer: The correct answer is (π+2)x(\pi + 2)x.
The perimeter of a semicircle is the sum of the curved part of the semicircle (half the circumference) and the diameter. The formula is πr+2r\pi r + 2r, where r=xr = x. This simplifies to (π+2)x(\pi + 2)x.
Quantitative Reasoning, Algebra, and Statistics- Question 13 of 20
Question: A hose can fill a cubical tank of edge length 1 meter in 15 minutes. At this rate, how long would it take for the hose to fill a cubical tank of edge length 2 meters?
Options:
- 12\frac{1}{2} hour
- 1 hour
- 1121\frac{1}{2} hours
- 2 hours
Answer: The correct answer is 2 hours.
The volume of the tank increases with the cube of the edge length. The volume of the larger tank is 8 times the smaller one (since 23=82^3 = 8), so it would take 8 times longer to fill. Since 15 minutes is 14\frac{1}{4} of an hour, 8×148 \times \frac{1}{4} hour = 2 hours.


Reviews
There are no reviews yet.